Multiplying Rational Expressions

After studying this lesson, you will be able to:

• Multiply rational expressions.

Steps to multiply a rational expression:

1. Cancel numerator to denominator if possible (don't cancel parts of a binomial or trinomial)

2. Factor the numerators and denominators if possible.

3. Multiply straight across - remember, you don't need a common denominator to multiply fractions (or rational expressions).

Example 1

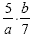	Nothing will cancel. Nothing will factor. All we have to do is multiply.
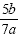	This is the simplified answer.

Example 2

	We can do some canceling and reducing in this problem. 2 and 16 reduces; 9 and 3 reduces, reduce the variables.
	Now, we multiply.
	This is the simplified expression.

Example 3

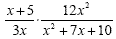	We can reduce 12 and 3 and reduce the variables
	Now, factor the second denominator.
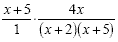	Cancel the identical binomials (x + 5 )
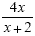	This is the simplified expression.

Example 4

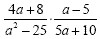	Factor
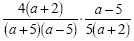	Cancel the identical binomials.
	This is the simplified expression.

Example 5

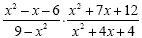	Factor
	Cancel the identical binomials.
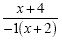	This is the simplified expression.